Imagine you live in a kingdom far, far away. It is a well-known and perhaps slightly overused fact that such kingdoms beget, in fact, tyrannical monarchs, ignorant despots, bigots of all sorts. It seems to be an implication woven into the very fabric of these realities, fueled by years of high fantasy lands dominated by all sorts of terrible men (and terrible writing).
These monarchs seem to be perpetually immersed in an illusion of grandeur, in the belief that the world will be a much better place if they get their ways. The ‘ways’ often involve rewriting the world from zero, starting from a clean slate. (To the members of my generation, this will strike as the recurring plot of evil villains from the Pokémon series). Today’s story speaks of a similar monarch, willing to bend reality, to forge (or perhaps force) a new universe to obtain their ultimate goal. Call them the monarch of the Kingdom of V.
Let us take a step back. Counting appears to us as such a basic skill that we very rarely stop to think about it. How do we recognize numbers? How do we know that four apples are less than five? The explanation seems to be way too complicated to be appropriately used as an answer to our question, invoking scary words like evolutionary psychology and neuroscience; and yet, the world of finite numbers seems harmless enough, at least from the mathematical perspective. If we accept that our world is made of sets, of collections, that we know at least one collection: the empty one, ∅. It is a set with zero elements. We can then form the collection that contains this set, {∅}, and know that it has precisely one element: ∅ itself. We can put these two together and form {{∅},∅}, a collection with two elements: {∅} and ∅. This bizarre construction can go on forever, giving rise to von Neumann ordinals. At least at the finite level, we can accept this as a construction of finite numbers. How do we know that a set X contains two elements? Well, we need to exhibit a correspondence, what is usually called a bijection, between the elements of X and the one set we know to have two elements, {{∅},∅}. We list the elements of X alongside the elements of {{∅},∅}, and if we get a precise correspondence we say that X has size, or cardinality, two.
This construction, albeit a bit convoluted, seems to satisfy our need for a definition of finite sizes, finite cardinalities. How about infinite sets? How do we measure, or count, the elements of infinite sets? The idea of a correspondence, of a bijection, seems to be fundamental: two sets have the same “number” of elements precisely if I can list them, one alongside the other, and no element is left out. Even if the process is infinite, this seems a satisfactory answer.
We may then turn to the infinite sets we know. The natural numbers are the first to come to mind: they are infinite — we cannot establish a correspondence with any finite ordinal as we have defined them — and yet feel very small. We call them countable. For example, they sit inside the real line, which is much bigger and denser than the natural one.
The reals themselves must be infinite, and yet they feel comparatively bigger than the natural numbers, as if there are more. This is true, and provable: the set of real numbers has more elements than the set of natural numbers. The former has the cardinality of the continuum.
Our intuition is powerful, but can lie to us; a similar train of thoughts could take us to believing that the rational numbers have more elements than the natural numbers. This is false: we can enumerate the rational numbers, and an explicit correspondence can be exhibited.
This leaves us slightly confused. We have different sizes of infinities, different so-called cardinalities, and we understand that some are bigger, and some are smaller. Yet our visual intuition is not very helpful: there are subsets of the real line which seem very different in size, and yet have the same cardinality. On the one side, we have countable subsets of the real line, like the natural, integer or rational numbers; on the other side, we have subsets with the cardinality of the continuum, like intervals, or the irrational numbers.
Is there anything in between?
Cantor, who dreamed this whole theory up (and later went mad), believed that the answer was no. This would be later called the Continuum Hypothesis (CH). He imagined a universe of sets that is well-behaved, under control, where there is no cardinality between the countable and the continuum. As the wisest among us could have told him, however, one should never meet their heroes; and, some ten years after his death, Cantor’s dream was shattered beyond repair.
Let us go back to the tyrannical ruler at the beginning of our story. They govern our universe of sets, with full powers and complete freedom. Rebelling against their gods, they seek to reach Cantor’s paradise and destroy it from within. They want to etch their name into eternity as the monarch who caused the death of gods, the failure of laws of reality. They want to bring into existence a contradiction to the Continuum Hypothesis, the equivalent of a law of physics that governs what their Kingdom should look like.
In the most classical path to hell, they enlist the help of many famous scientists, bright minds from all over the kingdom; they shall build a ladder to the heavens, from whence they will extract their much sought-after set whose cardinality will lie precisely between the countable and the continuum. The technicians get to work; the ladder starts rising up from the kingdom’s capitol, iron and wood and definable relations against the bright blue sky. Builders work night and day. They know how to reach the heavens, of course — there is only one direction: up.
Finally, after many years of work, the ladder touches the sky. A small door opens at the very end of the steps, carved from the fabric of the sky itself, a bronze handle awaiting the right hand, ‘V[G]’ etched in golden letters on the wood. The tyrant rushes up, and with a shudder — it is, in fact, very cold this high in the sky — they open the small door.
Behind it lies a counterexample to the Continuum Hypothesis, a set X with cardinality strictly bigger than countable and strictly smaller than continuum. The monarch erupts in triumph: they have succeeded! They have bent reality to their will!
What they have not realized, of course, is that they haven’t. Or in fact they have, but not quite how they imagined it. They have enlarged their Kingdom, their universe, to a new one, by opening the door that didn’t exist before; let us call it the Kingdom of V[G]. Even when expanded with only a small room, it is a radically different world from the one we are used to: it is a new reality, with different laws governing it. The most prominent example being, of course, the failure of the Continuum Hypothesis.
The monarch has managed to break a law of reality, a fundamental fact about the universe they used to live in; in doing so, however, they have forced into existence a new universe, a new world, with its own rules and facts. Nothing, of course, guarantees that the fact “the monarch rules over the Kingdom” has carried over from the Kingdom of V to the Kingdom of V[G]. This subtle point seems to have escaped our tyrant, for they didn’t spend much time in self-reflection. (This seems to be a common trait among villains).
This is a fairy tale, of course, albeit painted with the colours of true facts. Cantor would have never imagined that, with enough force of will and perhaps a bit of illusion of grandeur, we would have ever been able to construct universes out of other universes, bending their rules and laws to obtain what we want. He would have never expected that, through the technique of forcing we have just fairy-told our way through, the seemingly harmless statement of the Continuum Hypothesis would one day be proven independent of the axioms of Zermelo-Fraenkel, the basic rules of common sense that we expect our universes of sets to respect.
His paradise was shattered; many different copies of that paradise lie around, each one deformed to welcome a different truth, a different configuration of the world, a different view of reality. Many of them often rebel against their monarchs, against those who forced to be in that situation. After all, the existence of a monarch is not an absolute statement, and it most certainly does not follow from Zermelo-Fraenkel’s axioms. Through forcing we can bend reality, but we must always remember a crucial fact: any ladder that can be built can also be destroyed, and we’d better avoid being on top of it when that, inevitably, happens.



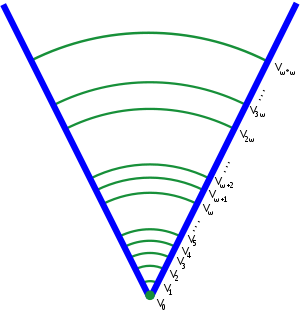
![A depiction of the Kingdom of V, together with the ladder, the door leading to the room where X is found, and the Kingdom of V[G] that contains the room. A depiction of the Kingdom of V, together with the ladder, the door leading to the room where X is found, and the Kingdom of V[G] that contains the room.](https://substackcdn.com/image/fetch/w_1456,c_limit,f_auto,q_auto:good,fl_progressive:steep/https%3A%2F%2Fbucketeer-e05bbc84-baa3-437e-9518-adb32be77984.s3.amazonaws.com%2Fpublic%2Fimages%2F5d8b4cec-0739-4cb4-8a58-0771ade30f01_1303x1129.png)